-
Samuel Velasco/Quanta Magazine -
A paper dodecahedron constructed in 2018 by David Aulicino and Jayadev Athreya to show that straight paths from a vertex back to itself while avoiding other vertices are in fact possible. (Patrick Hooper) -
Athreya’s right arm bears a tattoo of his favorite translation surface — a double pentagon. (Radhika Govindrajan)
Excerpt from article by Erica Klarreich | Quanta Magazine
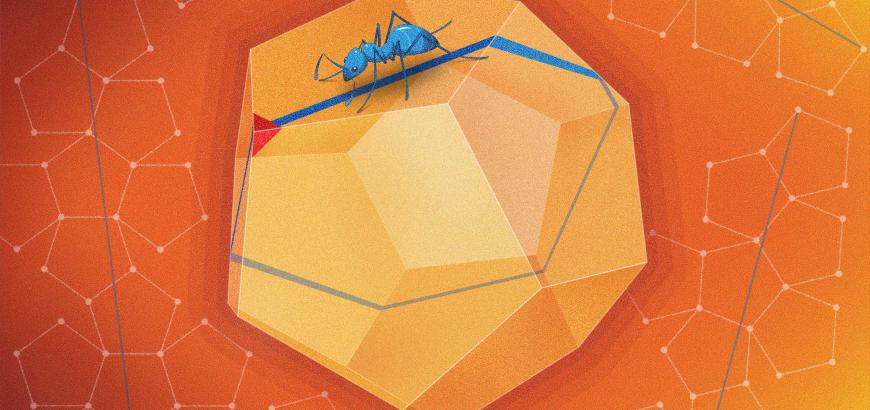
A trio of mathematicians has resolved one of the most basic questions about the dodecahedron. Jayadev Athreya, David Aulicino and Patrick Hooper have shown that an infinite number of such paths do in fact exist on the dodecahedron. Their paper, published in May in Experimental Mathematics, shows that these paths can be divided into 31 natural families.
The project began in 2016 when Athreya, of the University of Washington, and Aulicino, of Brooklyn College, started playing with a collection of card stock cutouts that fold up into the Platonic solids. As they built the different solids, it occurred to Aulicino that a body of recent research on flat geometry might be just what they’d need to understand straight paths on the dodecahedron. “We were literally putting these things together,” Athreya said. “So it was kind of idle exploration meets an opportunity.”
Together with Hooper, of the City College of New York, the researchers figured out how to classify all the straight paths from one corner back to itself that avoid other corners.
The analysis was “one of the most fun projects I’ve gotten to work on in my whole career,” Athreya said. “It’s important to keep playing with things.”
The new result shows that even objects that have been studied for thousands of years can still hold secrets, Eskin said. “I think even for [the three mathematicians], it was very, very surprising to say something new about the dodecahedron.”